Statistics Assignment Help With Bartlett's Test For Homogeneity
11.10 Bartlett's Test For Homogeneity Of Several Independent Estimates Of The Same Population Variance:
Let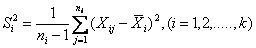 
Be the unbiased estimate of the population variance, obtained from the ith sample Xij,(j=1, 2, …….ni)and based on vi = (ni – 1) degrees if freedom, all the k samples being independent.
Under the null hypothesis that the samples come from the same population with variance σ2, that is the independent estimates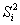 
, (i =1,2,….k) of σ2are homogeneous, Bartlet proved that the statistic
Where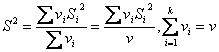 
Follows chi-square distribution with (k-1) degrees of freedom.
Email Based Assignment Help in Bartlett's Test For Homogeneity
To submit Bartlett's Test For Homogeneity assignment click here.
Following are some of the topics in Exact Sampling distributions in which we provide help:
- Exact Sampling distributions
- Derivation Of The Chi Square Distribution
- Moment Generating Function Of x2Distribution
- Cumulant Generating Function Of x2Distribution
- Limiting form of x2distribution for large degrees of freedom
- Characteristic function of x2distribution:
- Chi Square Probability Curve
- Conditions For The Validity Of Chi Square Test
Online Statistics Help | Statistics Math Help | Statistics probability help | Statistics help | College statistics help | Business statistics help | Elementary statistics help | Probability and statistics help | Statistics tutor | Statistic Homework help | Excel help | Mathematics Help | Matlab Help | MegaStat Help | Minitab help | PHStat2 Help | POM/QM help | R code and S-Plus help | SAS help | SPSS Help | Stata Help | TDISK Help | Tree Plan Help | Online Tutoring
