Probability Sample Assignment

41) (a) A Venn diagram

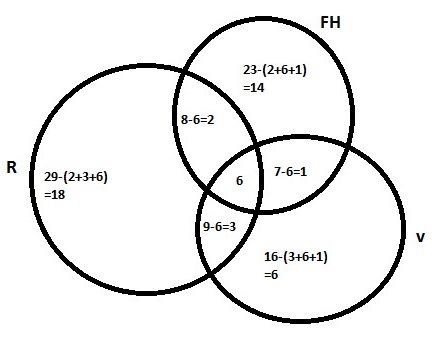
b) P(RuF) = P(R)+P(F)-P(R-F)

=29/50+23/50-8/50

=44/50

=0.88

c) 14+18+6=38

P(only one sport)

=38/50

= 0.76

d) P(Volleyball/Rugby)

=P(VuR)/P(R)

=9/29

=0.31

e) P(Field Hockey/Not Rugby)=15/21

=5/7

=0.714

35) (a) P(A’) and P(B’)

=7/100x13/100

=91/10000

(b) P(A) and P(B)

=93/100x87/100

=8091/10000

(c) At least one of the test detects steroid = 1-None shows presence of steroid

= 1-91/10000

=9909/10000

6) a) We have to fill 4 spaces. We are left with 8 numbers to choose from (1,2,3,4,5,6,8,9)

4P₄*7

7*5 ways – 5P₄8*7.

To eliminate zero, we fix zero in the first and rearrange the remaining number in the last four spaces. Hence, 4P₄*7 such cases,

The total codes = 5P₄*8*7)-4P₄*7

=280-28

=252 codes

1. b) 3 as the third and 5 as the fifth=5!/(n-2)!

=5x4x3x2x1/(5-2)!

=120/6

=20 Codes

1. c) To begin with an even number, The 1^st letter can be arranged in 4! Different forms starting with the four even numbers, that is 2,4,6,8

The 2^nd,3^rd, 4^th and 5^th can be arranged in 4! Ways. Hence, number of arrangement = 4!x4!

=576codes.

1. d) Begin and end with even number.

1^st – 4!

5^th – 4!

2^nd, 4^th and 5^th can be arranged into 3! ways.

Hence, The total codes=4!x4!x3!

=576x6

23) Let 5 be the 1st digit. The other four digits can have either of the other 9 choices, as we have excluded 8. This gives 9^4 numbers.

Let 5 be the 2nd digit. The first digit can have either of 7 choices, excluding 5, 8 and 0. The other three digits can have any of the 9 choices. This gives 7 * 9^3 numbers.

Let 5 be the 3^rd digit. The first digit can have either of 7 choices, excluding 5, 8 and 0. The 2^nd digit can have 8 choices excluding 5 and 8. The other two digits can have any of the 9 choices. This gives 7 * 8 *9^2 numbers.

Let 5 be the 4th digit. The first digit can have either of 7 choices, excluding 5, 8 and 0. The 2nd and 3rd digits can have any of 8 choices. This gives 7 * 8^2*9 numbers.

Let 5 be the 5th digit. The first digit can have either of 7 choices, excluding 5, 8 and 0. The 2nd, 3rd and 4th digits can have any of 8 choices. This gives 7 * 8^3 numbers.

Hence, the total possible numbers;

= 9^4 + 7*9^3 + 7*8*9^2 + 7*8^2*9 + 7*8^3= 23816